Why Atmospheric Temperature is a Linear Function of Mass & Gravity, and Not Influenced by Greenhouse Gas Concentrations

In the previous post of this series, we demonstrated why the US Standard Atmosphere Model & Observations Prove Maxwell's Mass/Gravity/Pressure Theory of the 'Greenhouse Effect' is Correct & Falsifies Anthropogenic Global Warming (CAGW).

We now show why the hundreds of rocket and atmospheric scientists, physicists, and aeronautical engineers who created the gold standard and final 1976 version of the US Standard Atmosphere Database (created during the ice age scare of the 1970's and just one decade prior to the global warming scare of the 1980's) in effect were "deniers" of any significant "radiative forcing," "heat trapping," or "radiative imbalance" from any greenhouse gases in their physical chemical calculations of the temperature profile of Earth's entire atmosphere from the surface all the way to the edge of space at ~100 kilometers altitude. 

In fact, the 241 page document provides overwhelming physical proof from physical chemistry and physics that the average annual temperatures at any altitude are controlled solely by molecular density, molecular weights, gravity, mass, pressure, etc. without any consideration of alleged "radiative forcing" or "heat trapping" from either natural or man-made CO2, nor any "radiative forcing" nor radiative considerations from any other gases including water vapor (now alleged to be the so-called 'primary greenhouse gas') whatsoever. The essential-to-CAGW claims of "radiative forcing," "heat trapping greenhouse gases," and "radiative imbalance from greenhouse gases" did not exist in 1976, and first appeared on the scene more than a decade later with James Hansen and the first IPCC 1990 report.

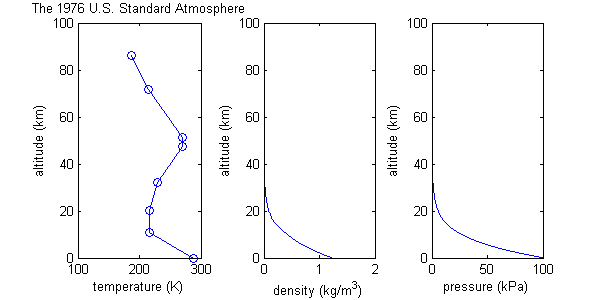
These pioneering atmospheric scientists calculated the effects of CO2 on the basis of the tiny 0.03-0.04% in the atmosphere (and thus contribution to molecular mass of the total atmosphere only ~0.03-0.04%) and found it to be so tiny and insignificant, that they removed CO2 from their 1-D model of the atmosphere completely. Their model was then used to calculate the US Standard Atmosphere database at every altitude from the surface to 100 km, and then overwhelmingly verified with millions of observations from weather balloons, research flights, rocket launches, etc. and found to accurately reproduce the temperatures on an annual basis at every altitude 0-120km within Earth's atmosphere, while completely omitting any mass, radiative, or any other effects from CO2 whatsoever.

In physics, the Stefan-Boltzmann equation is essential to calculate radiative emissions as a function of temperature (to the fourth power), but does not appear even one single time in any of the calculations in the 241 page US Standard Atmosphere description document or in their atmospheric model calculations. The Stefan-Boltzmann constant, which is absolutely essential to any radiative calculations from greenhouse gases or any gases or solid bodies also does not even appear once in the extensive tables of constants and definitions used in all of the calculations of the standard atmosphere, proving radiative considerations from any greenhouse gases are completely unnecessary to determine the average temperatures anywhere from the surface to the edge of space, and also that greenhouse gases have no radiative influence whatsoever upon the ~7 different lapse rates that occur in each of the ~7 different levels of the atmosphere from the surface to space. 

The Standard Atmosphere document indicates the only effect of water vapor upon the troposphere lapse rate is to reduce it from 9.8C/km to 6.5C/km on average, solely due to the high heat capacity Cp (1.865 Joules per gram per degree Kelvin) of water vapor compared to all of the other atmospheric gases. Per the lapse rate equation 

dT/dh = -g/Cp

where

dT = change in temperature with height
dh = change in height/geopotential altitude
g = gravitational acceleration constant = 9.8 meters/sec/sec
Cp = heat capacity at constant pressure (1 atmosphere constant pressure at the surface)

the temperature at any height or geopotential altitude is a function of and inversely related to heat capacity Cp. Thus any increase of Cp from water vapor will decrease the lapse rate and thus temperature at any height including at the surface (up to 25.5C as we previously calculated). This has absolutely nothing to do with "radiative forcing" from any greenhouse gases including water vapor itself. 

The first 6 of these linear lapse rates are shown in figure 3 below, and calculated entirely on the basis of geopotential altitude (a measure of gravitational potential energy PE) vs. molecular-scale temperature [defined in the scan below of page 9 as the mean molecular weight at that geopotential altitude], which has absolutely nothing to do with any alleged "heat trapping" or "radiative forcing" from any greenhouse gases:

Fig. 3 from the 1976 US Standard Atmosphere document below. Note the "Molecular-scale temperature is a function of the geopotential altitude." Thus, the kinetic temperature of the particular molecular masses and compositions of the atmosphere is a function of the geopotential height (which is the gravitational potential energy (PE) accumulated at that height) which adiabatically sets the pressure at that geopotential height. This is another way of saying temperature is a function of atmospheric mass/gravity/pressure, which is exactly what the Maxwell atmospheric mass/gravity/pressure 33C greenhouse effect claims, not "radiative forcing" from greenhouse gases. 

And we previous demonstrated with the greenhouse equation that we can exactly duplicate the 1976 US Standard Temperature database and model without even knowing anything about the surface temperature or greenhouse gases in advance, entirely based upon solar radiation at the Earth's surface, gravity, mass, and pressure of the atmosphere, proving no measurable effect from CO2. 

The "Greenhouse Equation" calculates temperature (T) at any location from the surface to the top of the troposphere as a function of atmospheric mass/gravity/pressure and radiative forcing from the Sun only, and without any radiative forcing from greenhouse gases. Note the pressure (P) divided by 2 in the greenhouse equation is the pressure at the center of mass of the atmosphere, where the temperature and height are equal to the equilibrium temperature with the Sun and average "Effective Radiating Level" or ERL, respectively. 

Fig 3 from the 1976 US Standard Atmosphere description document below, with added annotations showing how the center of the tropospheric lapse rate is "triangulated" by the known constants of mass and the center of mass, the height where the center of mass can be calculated which is where the mass above is 1/2 of the total mass and the Pressure (1/2 atm) is 1/2 of the surface pressure (1 atm), and the known constant of equilibrium temperature Te between the Earth and Sun. This is the exact location of the ERL, where the temperature must equal the equilibrium temperature with the Sun. By determining the height of the ERL, the greenhouse equation then extends the linear adiabatic lapse rate up to the top of the troposphere at ~12,000 meters and down to the surface at 0 meters, from which the entire tropospheric temperature profile from the top of the troposphere to the ERL to the surface temperature can be determined by only knowing the constant equilibrium temperature with the Sun = Te = 255K. 

Some commenters still doubt this is possible and conveniently claim [without any mathematical or observational proof whatsoever] as a last resort that greenhouse gases somehow control the lapse rates in each atmospheric layer. The US Standard Atmosphere and millions of confirming observations prove this is false, demonstrated by the linear kinematic velocity graph shown from the US Standard Atmosphere report below (from page 19 also scanned further below), which shows an almost perfect linear relationship between geopotential altitude and kinematic velocity from the surface to space, calculated entirely without any radiative forcing whatsoever and confirmed by observations. 

Greenhouse gas concentrations of the primary greenhouse gas water vapor and other greenhouse gases including methane, ozone, (and even CO2 to some extent) vary tremendously from the surface to the ~100 km edge of space, thus if "radiative forcing" from water vapor or any other greenhouse gases had anything to do with the cause of temperatures at any altitude, the kinematic viscosity would be nonlinear instead of linear, and the dynamic viscosity profile would not match the temperature profile (but the standard atmosphere shows it does). 

And the physical definitions and units below show radiance has nothing to do with any of these physical calculations nor interrelationships:

Physical definitions and units of kinematic viscosity, dynamic viscosity, mass density, weight and how they are related. None are defined on the basis of radiance or "radiative forcing"

This proves that only kinematic viscosity effects, not radiative effects, of any gases including greenhouse gases, are what determine the kinematic temperatures at all locations, not "greenhouse gas radiative forcing."  The only true source of radiative forcing is the Sun, not greenhouse gases which are mere passive IR-radiators & heat sinks, which help to cool the atmosphere by radiative loss to space, just like a bigger heat sink on your microprocessor does. 

After all of the above calculations were made by the US Std Atm scientists, the final calculation of the coefficient of thermal conductivity in W/(mK) was as their final step and determined solely as a function of geometric altitude/mass/density/viscosity. The document specifies below (page 20) that their ultimate calculation of the coefficient of thermal conductivity was the effect and not the cause of geometric altitude/mass/density/viscosity exclusively, and thus not "radiation trapping" nor any radiative calculations from any greenhouse gases whatsoever. The profile of these curves also nearly match the US Std Atmosphere temperature and dynamic viscosity graphs above indicating their direct relationship as gravity/mass the cause of and not the effect of any radiative forcing whatsoever.
Therefore, this provides the ultimate physical and observational proof that Maxwell's (33C) mass/pressure/gravity "greenhouse theory" of the (7) atmospheric temperature gradients is absolutely correct, thus falsifying the radiative greenhouse theory that is essential to the CAGW hypothesis. 

It is now absolutely clear that the greenhouse-gas radiative "greenhouse" theory has simply confused cause with effect. The Maxwell mass/gravity/pressure 33C "greenhouse theory" was proved physically and verified with the millions of observations of the 1976 US Standard atmosphere physical and modelled derivation and confirming observations, proving that temperatures everywhere from the surface to space are due to solar radiation plus the effect of atmospheric mass/gravity, thereby excluding any significant radiative effects from greenhouse gases (other than radiative cooling effects essential for the atmosphere to lose heat to space, i.e. the opposite of "trapping heat"). Only one of these two competing 33C "greenhouse effect" theories can be true, you simply cannot have it both ways, because if you did, the Earth would be 33C warmer at the surface than the present (in addition to multiple violations of physical laws). 

In our next post we will show why the radiation spectra of Earth seen from the ground and space are the effect of and not the cause of the entire atmospheric and surface profiles. 

CO2 and water vapor concentrations to number/mass density of the atmosphere are both so small they were calculated and then removed from consideration in the 1-D model of the atmosphere that perfectly reproduces the observations all the way to space.

1976 version of the US Standard Atmosphere

This is the most recent version and differs from previous versions only above 32 km:

Subscript b	Geopotential altitude above MSL[3]		Static pressure		Standard temperature (K)	Temperature Lapse Rate
	(m)	(ft)	(pascals)	(inHg)		(K/m)	(K/ft)
0	0	0	101325	29.92126	288.15	-0.0065	-0.0019812
1	11,000	36,089	22632.1	6.683245	216.65	0.0	0.0
2	20,000	65,617	5474.89	1.616734	216.65	0.001	0.0003048
3	32,000	104,987	868.019	0.2563258	228.65	0.0028	0.00085344
4	47,000	154,199	110.906	0.0327506	270.65	0.0	0.0
5	51,000	167,323	66.9389	0.01976704	270.65	-0.0028	-0.00085344
6	71,000	232,940	3.95642	0.00116833	214.65	-0.002	-0.0006096

The conventional radiative theory cannot explain why the linear lapse rates in each of the ~7 atmospheric layers defined in the US Std Atmosphere table above vary from positive to zero to negative on the basis of greenhouse gas radiative forcing, since the concentrations of the various greenhouse gases at each of these 7 levels varies tremendously in each level, often in the opposite direction to the concentrations of the composition and amounts of greenhouse gases in each level. It is frankly impossible for each greenhouse gas to magically have the opposite warming and cooling (or no) effects simply dependent upon the altitudes of the 7 different layers. In addition, the only greenhouse gases above level 4 above are CO2 and O3, which somehow are claimed by radiative proponents to magically warm the stratosphere and thermosphere, but cool the mesosphere. How can CO2 magically change it's radiative effects between layers of allegedly cooling 3 layers, having no effect on 3 layers, and the opposite warming effect on other layers? It cannot. CO2 and other greenhouse gases can only have the same passive IR absorbers/emitters in all 7 layers, increasing radiative surface area and thus cooling of the entire atmosphere to space.

Additional scans from the US Std Atmosphere proving all of the points above: