Why can't radiation from a cold body make a hot body hotter?

Three new posts by Quaternary geologist "okulaer" and astrophysicist Joe Postma further explain the misconceptions behind conventional greenhouse effect theory including

• Can radiation from a cold body increase the temperature of a warmer body?
• Are the Stefan-Boltzmann and Planck Laws applied correctly in calculating the greenhouse effect?
• How can radiation from a cold body not be thermalized [cause an increase in temperature] of a warmer body?
• How does quantum mechanics explain why a cold body can't make a warm body warmer still?
• How do photons "know" how to do this?
• Does water vapor warm or cool the planet?
• Do clouds warm or cool the planet?
• Why are cloudy nights warmer?
• Do clouds cause 25% of the greenhouse effect as claimed?
• In addition to the "back-radiation" misconceptions listed above and below, a prior HS post explains why global warming also cannot be explained by an increase of the "effective radiating level"

The real atmospheric greenhouse effect is instead entirely explainable on the basis of atmospheric mass/gravity/pressure/heat capacity/adiabatic lapse rate, and the "ocean greenhouse effect" explainable on the basis of the  ~0.76 - 0.89 far-IR emissivity of the oceans, which "traps" heat from solar radiation in the oceans.

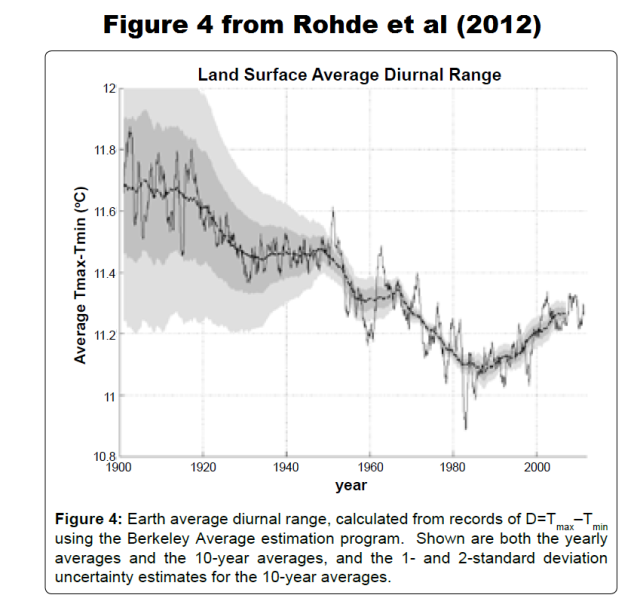
While water vapor and other greenhouse gases DO have a profound effect upon climate, the effect is a slowing of cooling at night and slowing of warming during the day [from evaporation, clouds, and enhancement of convection], resulting in a much more benign climate with decreased diurnal temperature range and decreased temperature extremes. 

Compared to the moon, which has a mean daytime temperature of 123C and mean nighttime temperature of -233C [a diurnal range of 356C!], the presence of Earth's atmosphere serves to greatly cool during the day and retain warmth during the night to reduce the diurnal temperature range to only ~11C. The global land diurnal temperature range has decreased over the past 110 years with the increase of greenhouse gases, as expected from a slowing of cooling at night and/or slowing of warming during the day.

In addition, a paper published in the Journal of Geophysical Research Atmospheres finds that daily [diurnal] temperature range in China decreased from 1962 to 2011, and that this decrease was due to a decrease in maximum temperatures related to a decrease of sunshine durations over this period. Similar to the findings of this paper, examination of the raw global temperature data [prior to tampering] shows a significant increase in minimum temperatures over the period 1962-1989, and then leveling off 1989-present. However, over the entire period 1940-present, there is no trend in minimum, maximum, or average global temperature anomalies. 

See two posts from Quaternary geologist okulaer "The greenhouse effect that wasn't" Part 1 and Part 2

Excerpts from Climate of Sophistry by Joe Postma, emphasis/bolding added:

Why not Backradiation? The Amazing Nature of Light

Posted on 2014/11/16 Climate of Sophistry, emphasis addedby Joseph E Postma

The Basics

It seems that a major source of confusion stems around this equation for radiative transfer of heat energy:

Q = σT24 – σT14

The term ‘Q’ is not the incoming solar energy nor does it represent a source of energy at all. From that incorrect interpretation of the equation arises all sorts of further misinterpretations and bad physics.  It’s where the whole incorrect idea of backradiation heating arises and all of the various arguments about cold helping to make something warmer hotter still.  I address that misinterpretation of the equation many times on this blog, but here I do it up front:

http://climateofsophistry.com/2013/12/08/revisiting-the-steel-greenhouse/

‘Q’ is the heat flow between the Sun and Earth and so is not the solar energy.  The solar energy flux would be a term on the right hand side, σT24 say, but factored for distance. How this is done is demonstrated in the link above.  ‘Q’ is actually zero if we consider the Earth to be in energy equilibrium with the solar input, which it should be within a small margin.

It is also discussed here:

http://climateofsophistry.com/2013/05/27/the-fraud-of-the-aghe-part-12-how-to-lie-with-math/

So to repeat, ‘Q’ can not be the solar heat input, when T1 and T2 are supposed to be the temperatures of the atmosphere and surface. That’s not what that equation is about at all.

I’ll let Rosco explain, and I was going to blockquote his comment from a previous thread but with my own editing now finished, I’ll just acknowledge that this next section comes largely from him:

The Physics

‘Q’ is not an actual radiant emission corresponding to any temperature and hence is not subject to any valid algebraic manipulation as if it supposed to be a conserved quantity from some source.  It is not a conserved quantity and it does not represent a source.

‘Q’  is actually Q(net) – the difference between two emission powers from 2 objects.

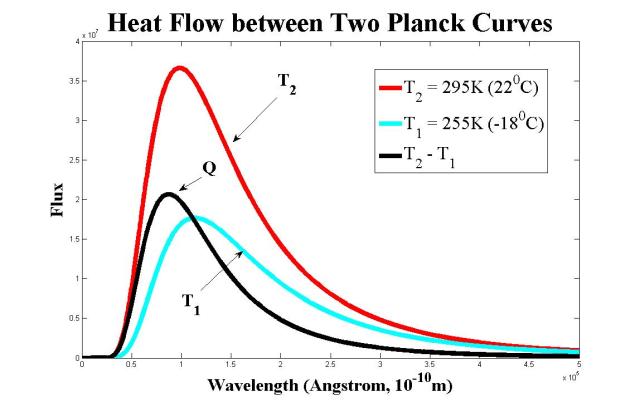
This is easily observed on a Planck curve diagram.

σT14 is the area between the Planck curve and the x axis for T1.

σT24 is the area between the Planck curve and the x axis for T2. If T2 > T1 then the area of the Planck curve for T2 completely includes all of σT14.

But ‘Q’ = Q(net) = σT24 – σT14 is not a radiative flux at all. It is the area between the T2 Planck curve and the T1 Planck curve!

Only the T1 and T2 terms have any relationship to the Stefan-Boltzmann equation because they are explicitly derived from Planck’s equation as the integral from 0 to infinity of Planck’s equation in either df (frequency) or dlambda (wavelength) terms.

If T1 = T2 then Q(net) = 0 this simply says that two objects at the same temperature have zero net energy exchange and therefore no thermal effect on each other.

If T1 is less than T2 then object 2 is heating object 1 and raising its temperature. This demonstrates the ridiculous nature of all “greenhouse effect” “physics” – they simply play algebraic tricks without any recognition of what the terms actually mean.

F1 = σT14 is a valid flux for T1; F1 = σT14 is a valid flux for T2.

Q = σT24 – σT14 is just a number, just the difference of the fluxes – nothing more! It is not a source in itself.  It is not conserved.  You can’t hold it constant and say that an increase in the cooler T1 will cause an increase in the warmer T2, i.e., that cold can heat hot.

To manipulate this expression by algebra and claiming that ‘Q’ has to remain fixed because it is the energy from the Sun is entirely nonsensical by the terms and logic of the equation itself.

The Question

So this is the question that confuses just about everybody, and it is also where the error of backradiation heating and the misinterpretation of the heat flow equation originates:

What happens to the energy from the cooler portion if it travels to the hotter side but does nothing?  How can it do nothing?  Does it even travel to the hotter side at all?  How can it not?  How could it know not to?  How could the photons from the cooler side either A) not cause any heating when they get to the hotter side, or B) not travel to the other side at all?  These are all related questions.

To be sure, the equation we’ve been discussing for ‘Q’ absolutely, most definitely, 100% says that radiative heat energy only goes from hot to cold and thus that temperature can only be increased by something hot warming up something cooler.  It specifically does not result in something like the supposed “steel greenhouse effect”, as debunked previously.  And this equation is the correct equation from radiative transfer theory and thermodynamics, when used and interpreted properly.  It says what happens, and so it appears that the problem is that it doesn’t say why that happens.

The Answer

The radiation from the cooler source doesn’t have the high frequency light-wave energy components which would be required to fill up the higher-energy microstates that the warmer source already has filled up, let alone the higher frequency states beyond to result in an increase in temperature.  You can see it a little bit in the Planck curve plot above, that the higher-temperature object has a microstate population that is “activated” at higher frequencies, i.e. shorter/smaller wavelengths, than the lower-temperature object has.  (The warmer one also has a larger population in each frequency microstate as compared to the cooler one.)

The integration over all the active microstates of a system determines its macrostate, and the macrostate is the thing you actually measure to take a temperature.  If you activate higher-frequency microstates, then you shift the macrostate to a higher temperature.  But you can only activate the higher-frequency microstates with the frequencies required to activate them, which are obviously their frequencies.

And so because the radiation from a cooler source lacks the higher-frequency microstates that the warmer source of radiation already has, then the cooler source can not have the effect of raising the temperature of the warmer source.  And obviously the same would be true of two systems with identical temperatures – they could have no effect on each other’s microstate population, hence can not heat each other.

For example, if we add the two Planck thermal radiation curves from the above plot together, then the existing microstate populations are increased, but it doesn’t activate any new higher-frequency microstates than the warmer curve already had activated, and thus the temperature doesn’t get increased:

Now you can’t see it because the lines all overlap each other at high energy (i.e. short/small wavelength), but the cooler curve has zero contribution, zero activated microstates, at the high energy, high frequency, short wavelength end of the plot (left side) where the warmer curve does have populated microstates.  Thus, adding the cooler radiation with the warmer radiation doesn’t result in a macrostate that has microstates activated at higher frequencies as compared to the original warmer radiation (T2), and thus, the result doesn’t have a higher temperature than the original warmer source of radiation .

Also note, and this you can see, that the peak of the population of microstates for the sum of the warm and cool source radiation is at longer wavelength and thus lower energy than the original warm source radiation.  Adding cool to hot doesn’t result in something hotter…but adding hot to cool certainly does result in something hotter.

But still the question can be asked:  Why doesn’t the addition of energy from the cooler source to the warmer source result in an increase in temperature for the warmer source?  If you add any energy to anything, doesn’t that have to result in an increase in internal energy, and thus temperature?

The answer to that, is “No”, because that is not how thermodynamics works.  It’s not what the radiative heat transfer equation says or how it works.

It is not that simple.

More Answer

We actually shouldn’t have add those fluxes together at all because that is not how heat radiatively transfers.  If we add those fluxes, then is that now the flux that the hotter object emits, or is it the cooler objects?  Or is that the radiation field between the two objects?  None of those are correct.  It was wrong to add those fluxes together.  It is easy to make that mistake, but on the other hand, we’ve always had the equation for radiative heat transfer at hand and so we should simply refer to it and obey it.  The energy field flowing as heat between the hot and cool objects is only ‘Q’, only the difference between the hot and cool fluxes.

You can see a little more clearly now that ‘Q’, the heat flow, merges to the hotter curve at high frequency/small wavelength, which again is all about how only the hotter source has active microstates at high frequency, and the cooler curve doesn’t.  If you add the ‘Q’ curve, the heat flow, to the T1 object’s curve, then you get the T2 curve, which would indicate that object 1 has come to the temperature of object 2 in thermal equilibrium, and then the heat flow ‘Q’ would be zero, meaning that no more temperature changes can occur.  Object 1 & 2 become a unified thermal system on the side facing each other, and object 1 then simply emits the energy supplied by object 2 out from the side facing way from object 2.

When you have say, two planes of material, touching each other, then the heat flow would be purely diffusive, meaning purely by conduction.  The addition of another layer physically touching the existing warmer layer doesn’t make the warmer layer warmer still – the new layer simply gets heated to the temperature of the original layer, and then it becomes the new surface of the original warmer plane.  Nothing about this changes when there is a gap between the layers so that the heat transfer between them become radiative.  It’s not like you would remove the added layer from touching the original one to create a vacuum gap in between, and suddenly this would cause the warmer layer to become warmer still, when this is not what happened when the layers were touching.  The modes of heat transfer obey the same limitations and the same general rules of heat flow.

The situation for the Sun and Earth is a little different.  Scaled for local intensity at the distance of the Earth from the Sun, the solar spectrum has much less intensity at terrestrial frequencies and thus the Earth can locally shed heat energy in the direction of the Sun.  You can see this in idealized Planck curves given the solar and terrestrial effective temperatures:

The Solar and Terrestrial fluxes are scaled for distance of the Sun from Earth, and for 4-times the emission from a sphere for the Earth as compared to the disk cross-section of absorption.

However, as we calculated in the steel greenhouse debunking thread, the radius of heat potential for the Earth only extends to 56.8 million kilometers before the terrestrial heat becomes indistinguishable from the cosmic background thermal radiation.  Therefore, the Earth can not heat the Sun with terrestrial backradiation (because the Sun is 150 million kilometers distant), and that will always be a general result in any scenario.

Of course, as you go back towards the Sun, the solar flux spectrum increases in intensity and so well before reaching the Sun, the flux from the Earth will become dominated by the outward flux from the Sun at those wavelengths.  The flux intensities from the Earth vs. the Sun, at the wavelength of the peak of the terrestrial spectrum, become equal at 27.1 million kilometers distance from the Earth towards the Sun, which is well inside the heat envelope of Earth.

 Final Consideration

All of the GHE advocates make this same mistake of misinterpreting the meaning of the terms of radiative heat transfer equation.  They all have this idea that ‘Q’ is a conserved quantity as if is the energy flux from the Sun.  This is wrong.  How surprised would they be if they realized that ‘Q’ was actually zero, given that we generally assume a state of thermal equilibrium between the solar input and terrestrial output, thus requiring that Q equals zero?

Furthermore, if they make such a basic mistake with such a simple and basic equation, then how can you really trust the rest of what they’re doing?  This is first-year undergraduate level stuff, and not only does it seem entirely beyond them, they actually get extremely angry and hostile when you ask them to read up on it and correct such a simple mistake.

But we have one last amazing thing to think about with regard to radiation.  Sure the stuff about energy microstates and thermodynamic macrostates is all very interesting, and describes what happens to some degree, but still people may wonder:  Why do those fluxes not add together if their energy is in the same region of space?  What happens to the radiative energy if only the difference, not the sum, has an effect on inducing temperature changes?  How do the photons “know” how to do this?

Well firstly, that is exactly how vectors behave.  This Wiki article on heat transfer physics is a little mathematically advanced, but the term we’ve been denoting as ‘Q’ here is a “heat flux vector”.  Opposing vectors subtract, not add.

And finally, think of the way that the universe is experienced by a photon.  A photon travels at the speed of light and so time is infinitely dilated and space is infinitely shrunk.  A photon quite literally exists outside of space and time as we know it.  It doesn’t experience time, and it doesn’t experience space!

Just apply the Lorentz relativity equations with the subject in question being a photon, traveling at the speed of light ‘c‘.  The equations directly say that neither time nor space is experienced by a photon.  A photon has no distance to travel as far as it is concerned, since there is no space because all spatial length is infinitely contracted, and it has no time to experience because time has come to a complete stand-still with infinite time dilation.

So a photon has no distance to travel, in no time – from its perspective, and we have to grant it its own perspective as per relativity theory.  And so, under these conditions, a photon essentially does know what its destination is like and so radiative transfer of heat energy can be limited to the same rules as physical-contact flows such as with conduction.

How does a photon from a cool spectrum source “know” not to travel to and warm up a warmer source?  It is because a photon is effectively outside of space and time.

Start thinking of what life as a photon must be like, if you were a photon, travelling at the speed of light, and wrap your head around that.